This method uses trigonometric identities and Helen’s Formula

Given \cos A + 2 \cos B + \cos C = 2

⇒ \cos A + \cos C = 2(1 – \cos B)

Using Sum to Product Identity

\cos \alpha + \cos \beta = 2\cos\dfrac{\alpha +\beta }{2} \cos\dfrac{\alpha -\beta }{2}

and Double angle identity

\cos2 \alpha = 1-2 \sin^2 \alpha

⇒ 2 \cos\dfrac{A+C}{2} \cdotp \cos\dfrac{A-C}{2} = 4 \sin^2(\dfrac{B}{2} )

Since the sum of the three internal angles is equal to \pi

\therefore \dfrac{A+C}{2} = \dfrac{\pi}{2}-\dfrac{B}{2}

⇒ 2 \sin\dfrac{B}{2}\cos\dfrac{A-C}{2} = 4\sin^2 \dfrac{B}{2}

⇒ \cos\dfrac{A-C}{2} – \cos\dfrac{A+C}{2} = \cos\dfrac{A+C}{2}

Using Helen formula

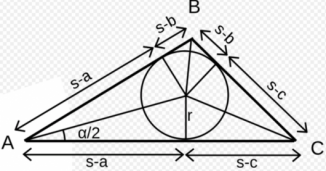
\because A =\dfrac{1}{2} (a+b+c)r

r = \dfrac{A}{s}

= \dfrac{ \sqrt{s(s-a)(s-b)(s-c)}}{s}

=\sqrt{\dfrac{(s-a)(s-b)(s-c)}{s} }

in which A is the area of the triangle, s = \dfrac{a+b+c}{2}

\sin \dfrac{A}{2} =\dfrac{r}{\sqrt{r^2+(s-a)^2} }

=\dfrac{1}{\sqrt{1+\dfrac{(s-a)^2}{r^2} } }

=\dfrac{1}{\sqrt{1+\dfrac{s(s-a)}{(s-b)(s-c)} } }

=\dfrac{\sqrt{(s-b)(s-c)} }{\sqrt{s^2-(b+c)s+bc+s^2-sa}}

=\dfrac{\sqrt{(s-b)(s-c)} }{\sqrt{2s^2-(a+b+c)s+bc}}

=\dfrac{\sqrt{(s-b)(s-c)} }{\sqrt{bc}}

Similarly,

\sin \dfrac{B}{2} = \dfrac{\sqrt{(s-a)(s-c)} }{\sqrt{ac}}

\sin \dfrac{C}{2} = \dfrac{\sqrt{(s-a)(s-b)} }{\sqrt{ab}}

Substituting to (2) yields

⇒ 2(s – b) = b

⇒ a + b + c – 2b = b

⇒ a + c = 2b

This method uses trigonometric identities and the Law of Sines

Following (1) of the method 1

Multiplying 2\cos \dfrac{B}{2} with two sides of the equation

2\cos\dfrac{A-C}{2} \cos \dfrac{B}{2} = 2\cdotp 2\sin\dfrac{B}{2}\cos \dfrac{B}{2}

2\cos\dfrac{A-C}{2} \sin \dfrac{A+C}{2} = 2\cdotp 2\sin B

Using Product to sum identity,

\cos \alpha\sin \beta = \dfrac{1}{2} [\sin(\alpha+\beta ) - \sin(\alpha - \beta) ]

Using the Law of Sines,

\sin A=\dfrac{a}{2R}

\sin B=\dfrac{b}{2R}

\sin C=\dfrac{c}{2R}

Substituting to (2) yields

2b=a+c